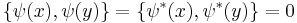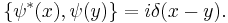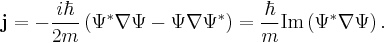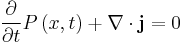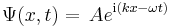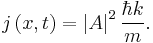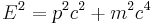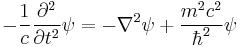Schrödinger equation
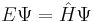 |
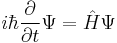 |
| Two forms of the Schrödinger equation |
| Quantum mechanics | ||||||||||||||||
 |
||||||||||||||||
| Uncertainty principle |
||||||||||||||||
Introduction · Mathematical formulations
|
||||||||||||||||
In physics, specifically quantum mechanics, the Schrödinger equation, formulated by Austrian physicist Erwin Schrödinger, is an equation that describes how the quantum state of a physical system changes in time. It is as central to quantum mechanics as Newton's laws are to classical mechanics.
In the standard interpretation of quantum mechanics, the quantum state, also called a wavefunction or state vector, is the most complete description that can be given to a physical system. Solutions to Schrödinger's equation describe not only molecular, atomic and subatomic systems, but also macroscopic systems, possibly even the whole universe. The equation is named after Erwin Schrödinger, who constructed it in 1926.[1]
The most general form is the time-dependent Schrödinger equation, which gives a description of a system evolving with time. For systems in a stationary state, the time-independent Schrödinger equation is sufficient. Approximate solutions to the time-independent Schrödinger equation are commonly used to calculate the energy levels and other properties of atoms and molecules.
Schrödinger's equation can be mathematically transformed into Werner Heisenberg's matrix mechanics, and into Richard Feynman's path integral formulation. The Schrödinger equation describes time in a way that is inconvenient for relativistic theories, a problem which is not as severe in matrix mechanics and completely absent in the path integral formulation.
Contents |
The Schrödinger equation
The Schrödinger equation takes several different forms, depending on the physical situation. This section presents the equation for the general case and for the simple case encountered in many textbooks.
General quantum system
For a general quantum system:[2]
where
-
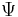 is the wave function; the probability amplitude for different configurations of the system at different times,
is the wave function; the probability amplitude for different configurations of the system at different times, is the energy operator (
is the energy operator ( is the imaginary unit and
is the imaginary unit and  is the reduced Planck constant),
is the reduced Planck constant),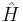 is the Hamiltonian operator.
is the Hamiltonian operator.
Single particle in a potential
For a single particle with potential energy V, the Schrödinger equation takes the form:[3]
where
-
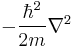 is the kinetic energy operator, where m is the mass of the particle.
is the kinetic energy operator, where m is the mass of the particle.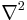 is the Laplace operator. In three dimensions, the Laplace operator is
is the Laplace operator. In three dimensions, the Laplace operator is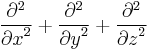 , where x, y, and z are the Cartesian coordinates of space.
, where x, y, and z are the Cartesian coordinates of space. is the time-independent potential energy at the position r.
is the time-independent potential energy at the position r.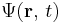 is the probability amplitude for the particle to be found at position r at time t.
is the probability amplitude for the particle to be found at position r at time t.
Time independent equation
The time independent equation, again for a single particle with potential energy V takes the form:[4]
This equation describes the standing wave solutions of the time-dependent equation, which are the states with definite energy.
Historical background and development
Following Max Planck's quantization of light (see black body radiation), Albert Einstein interpreted Planck's quantum to be photons, particles of light, and proposed that the energy of a photon is proportional to its frequency, one of the first signs of wave–particle duality. Since energy and momentum are related in the same way as frequency and wavenumber in special relativity, it followed that the momentum p of a photon is proportional to its wavenumber k.
Louis de Broglie hypothesized that this is true for all particles, even particles such as electrons. Assuming that the waves travel roughly along classical paths, he showed that they form standing waves for certain discrete frequencies. These correspond to discrete energy levels, which reproduced the old quantum condition.[5]
Following up on these ideas, Schrödinger decided to find a proper wave equation for the electron. He was guided by William R. Hamilton's analogy between mechanics and optics, encoded in the observation that the zero-wavelength limit of optics resembles a mechanical system—the trajectories of light rays become sharp tracks which obey Fermat's principle, an analog of the principle of least action.[6] A modern version of his reasoning is reproduced in the next section. The equation he found is:
Using this equation, Schrödinger computed the hydrogen spectral series by treating a hydrogen atom's electron as a wave Ψ(x, t), moving in a potential well V, created by the proton. This computation accurately reproduced the energy levels of the Bohr model.
However, by that time, Arnold Sommerfeld had refined the Bohr model with relativistic corrections.[7][8] Schrödinger used the relativistic energy momentum relation to find what is now known as the Klein–Gordon equation in a Coulomb potential (in natural units):
He found the standing waves of this relativistic equation, but the relativistic corrections disagreed with Sommerfeld's formula. Discouraged, he put away his calculations and secluded himself in an isolated mountain cabin with a lover.[9]
While at the cabin, Schrödinger decided that his earlier non-relativistic calculations were novel enough to publish, and decided to leave off the problem of relativistic corrections for the future. He put together his wave equation and the spectral analysis of hydrogen in a paper in 1926.[10] The paper was enthusiastically endorsed by Einstein, who saw the matter-waves as an intuitive depiction of nature, as opposed to Heisenberg's matrix mechanics, which he considered overly formal.[11]
The Schrödinger equation details the behaviour of ψ but says nothing of its nature. Schrödinger tried to interpret it as a charge density in his fourth paper, but he was unsuccessful.[12] In 1926, just a few days after Schrödinger's fourth and final paper was published, Max Born successfully interpreted ψ as a probability amplitude.[13] Schrödinger, though, always opposed a statistical or probabilistic approach, with its associated discontinuities—much like Einstein, who believed that quantum mechanics was a statistical approximation to an underlying deterministic theory— and never reconciled with the Copenhagen interpretation.[14]
Derivation
Short heuristic derivation
Schrödinger's equation can be derived in the following short heuristic way.
Assumptions
- The total energy E of a particle is
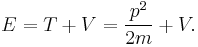
- This is the classical expression for a particle with mass m where the total energy E is the sum of the kinetic energy T, and the potential energy V (which can vary with position, and time). p and m are respectively the momentum and the mass of the particle.
- Einstein's light quanta hypothesis of 1905, which asserts that the energy E of a photon is proportional to the frequency ν (or angular frequency, ω = 2πν) of the corresponding electromagnetic wave:
- The de Broglie hypothesis of 1924, which states that any particle can be associated with a wave, and that the momentum p of the particle is related to the wavelength λ (or wavenumber k) of such a wave by:

- Expressing p and k as vectors, we have
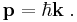
- The three assumptions above allow one to derive the equation for plane waves only. To conclude that it is true in general requires the superposition principle, and thus, one must separately postulate that the Schrödinger equation is linear.
Expressing the wave function as a complex plane wave
Schrödinger's idea was to express the phase of a plane wave as a complex phase factor:
and to realize that since
then
and similarly since
and
we find:
so that, again for a plane wave, he obtained:
And, by inserting these expressions for the energy and momentum into the classical formula we started with, we get Schrödinger's famed equation, for a single particle in the 3-dimensional case in the presence of a potential V:
Versions
There are several equations that go by Schrödinger's name:
Time dependent equation
This is the equation of motion for the quantum state. In the most general form, it is written:[15]
where 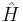 is a linear operator acting on the wavefunction Ψ. For the specific case of a single particle in one dimension moving under the influence of a potential V.[15]
is a linear operator acting on the wavefunction Ψ. For the specific case of a single particle in one dimension moving under the influence of a potential V.[15]
and the operator  can be read off:
can be read off:
For a particle in three dimensions, the only difference is more derivatives:
and for N particles, the difference is that the wavefunction is in 3N-dimensional configuration space, the space of all possible particle positions.[16]
This last equation is in a very high dimension, so that the solutions are not easy to visualize.
Time independent equation
This is the equation for the standing waves, the eigenvalue equation for 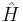 . In abstract form, for a general quantum system, it is written:[15]
. In abstract form, for a general quantum system, it is written:[15]
For a particle in one dimension,
But there is a further restriction—the solution must not grow at infinity, so that it has a either a finite L2-norm (if it is a bound state) or a slowly diverging norm (if it is part of a continuum):[17]
For example, when there is no potential, the equation reads:[18]
which has oscillatory solutions for E > 0 (the Cn are arbitrary constants):
and exponential solutions for E < 0
The exponentially growing solutions have an infinite norm, and are not physical. They are not allowed in a finite volume with periodic or fixed boundary conditions.
For a constant potential V the solution is oscillatory for E > V and exponential for E < V, corresponding to energies which are allowed or disallowed in classical mechanics. Oscillatory solutions have a classically allowed energy and correspond to actual classical motions, while the exponential solutions have a disallowed energy and describe a small amount of quantum bleeding into the classically disallowed region, to quantum tunneling. If the potential V grows at infinity, the motion is classically confined to a finite region, which means that in quantum mechanics every solution becomes an exponential far enough away. The condition that the exponential is decreasing restricts the energy levels to a discrete set, called the allowed energies.
Nonlinear equation
The nonlinear Schrödinger equation is the partial differential equation (in dimensionless form)[19]
for the complex field ψ(x,t).
This equation arises from the Hamiltonian[19]
with the Poisson brackets
It must be noted that this is a classical field equation. Unlike its linear counterpart, it never describes the time evolution of a quantum state.
Properties
The Schrödinger equation has certain properties.
Local conservation of probability
The probability density of a particle is 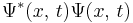 . The probability flux is defined as [in units of (probability)/(area × time)]:
. The probability flux is defined as [in units of (probability)/(area × time)]:
The probability flux satisfies the continuity equation:
where 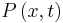 is the probability density [measured in units of (probability)/(volume)]. This equation is the mathematical equivalent of the probability conservation law.
is the probability density [measured in units of (probability)/(volume)]. This equation is the mathematical equivalent of the probability conservation law.
For a plane wave:
So that not only is the probability of finding the particle the same everywhere, but the probability flux is as expected from an object moving at the classical velocity p/m. The reason that the Schrödinger equation admits a probability flux is because all the hopping is local and forward in time.
Relativity
The Schrödinger equation does not take into account relativistic effects; as a wave equation, it is invariant under a Galilean transformation, but not under a Lorentz transformation. But in order to include relativity, the physical picture must be altered.
The Klein–Gordon equation uses the relativistic mass-energy relation:
to produce the differential equation:
which is relativistically invariant.
Solutions
Some general techniques are:
- Perturbation theory
- The variational method
- Quantum Monte Carlo methods
- Density functional theory
- The WKB approximation and semi-classical expansion
In some special cases, special methods can be used:
- List of quantum-mechanical systems with analytical solutions
- Hartree-Fock method and post Hartree-Fock methods
- Discrete delta-potential method
See also
- Basic concepts of quantum mechanics
- Dirac equation
- Quantum chaos
- Quantum number
- Relation between Schrödinger's equation and the path integral formulation of quantum mechanics
- Schrödinger's cat
- Schrödinger field
- Schrödinger picture
- Theoretical and experimental justification for the Schrödinger equation
Notes
- ↑ Schrödinger, E. (1926). "An Undulatory Theory of the Mechanics of Atoms and Molecules". Physical Review 28 (6): 1049–1070. doi:10.1103/PhysRev.28.1049. http://home.tiscali.nl/physis/HistoricPaper/Schroedinger/Schroedinger1926c.pdf.
- ↑ Shankar, R. (1994). Principles of Quantum Mechanics (2nd ed.). Kluwer Academic/Plenum Publishers. p. 143. ISBN 978-0-306-44790-7.
- ↑ Shankar, R. (1994). Principles of Quantum Mechanics (2nd ed.). Kluwer Academic/Plenum Publishers. p. 143ff. ISBN 978-0-306-44790-7.
- ↑ Shankar, R. (1994). Principles of Quantum Mechanics (2nd ed.). Kluwer Academic/Plenum Publishers. p. 145. ISBN 978-0-306-44790-7.
- ↑ de Broglie, L. (1925). "Recherches sur la théorie des quanta [On the Theory of Quanta]". Annales de Physique 10 (3): 22–128. http://tel.archives-ouvertes.fr/docs/00/04/70/78/PDF/tel-00006807.pdf. Translated version.
- ↑ Schrodinger, E. (1984). Collected papers. Friedrich Vieweg und Sohn. ISBN 3700105738. See introduction to first 1926 paper.
- ↑ Sommerfeld, A. (1919). Atombau und Spektrallinien. Braunschweig: Friedrich Vieweg und Sohn. ISBN 3871444847.
- ↑ For an English source, see Haar, T.. The Old Quantum Theory.
- ↑ Rhodes, R. (1986). Making of the Atomic Bomb. Touchstone. ISBN 0-671-44133-7.
- ↑ Schrödinger, E. (1926). "Quantisierung als Eigenwertproblem; von Erwin Schrödinger". Annalen der Physik, (Leipzig): 361–377. http://gallica.bnf.fr/ark:/12148/bpt6k153811.image.langFR.f373.pagination.
- ↑ Einstein, A.; et. al.. Letters on Wave Mechanics: Schrodinger-Planck-Einstein-Lorentz.
- ↑ Moore, W.J. (1992). Schrödinger: Life and Thought. Cambridge University Press. p. 219. ISBN 0-521-43767-9.
- ↑ Moore, W.J. (1992). Schrödinger: Life and Thought. Cambridge University Press. p. 220. ISBN 0-521-43767-9.
- ↑ It is clear that even in his last year of life, as shown in a letter to Max Born, that Schrödinger never accepted the Copenhagen interpretation. cf p. 220 Moore, W.J. (1992). Schrödinger: Life and Thought. Cambridge University Press. p. 479. ISBN 0-521-43767-9.
- ↑ 15.0 15.1 15.2 Shankar, R. (1994). Principles of Quantum Mechanics. Kluwer Academic/Plenum Publishers. pp. 143ff. ISBN 978-0-306-44790-7.
- ↑ Shankar, R. (1994). Principles of Quantum Mechanics. Kluwer Academic/Plenum Publishers. p. 141. ISBN 978-0-306-44790-7.
- ↑ Feynman, R.P.; Leighton, R.B.; Sand, M. (1964). "Operators". The Feynman Lectures on Physics. 3. Addison-Wesley. pp. 20–7. ISBN 0201021153.
- ↑ Shankar, R. (1994). Principles of Quantum Mechanics. Kluwer Academic/Plenum Publishers. pp. 151ff. ISBN 978-0-306-44790-7.
- ↑ 19.0 19.1 V.E. Zakharov; S.V. Manakov (1974). "On the complete integrability of a nonlinear Schrödinger equation". Journal of Theoretical and Mathematical Physics 19 (3): 551–559. doi:10.1007/BF01035568. Originally in: Teoreticheskaya i Matematicheskaya Fizika 19 (3): 332–343. June, 1974
References
- Paul Adrien Maurice Dirac (1958). The Principles of Quantum Mechanics (4th ed.). Oxford University Press.
- David J. Griffiths (2004). Introduction to Quantum Mechanics (2nd ed.). Benjamin Cummings. ISBN 0131244051.
- Richard Liboff (2002). Introductory Quantum Mechanics (4th ed.). Addison Wesley. ISBN 0805387145.
- David Halliday (2007). Fundamentals of Physics (8th ed.). Wiley. ISBN 0471159506.
- Serway, Moses, and Moyer (2004). Modern Physics (3rd ed.). Brooks Cole. ISBN 0534493408.
- Walter John Moore (1992). Schrödinger: Life and Thought. Cambridge University Press. ISBN 0521437679.
- Schrödinger, Erwin (December 1926). "An Undulatory Theory of the Mechanics of Atoms and Molecules". Phys. Rev. 28 (6) 28: 1049–1070. doi:10.1103/PhysRev.28.1049.
External links
- Quantum Physics - a textbook with a treatment of the time-independent Schrödinger equation
- Linear Schrödinger Equation at EqWorld: The World of Mathematical Equations.
- Nonlinear Schrödinger Equation at EqWorld: The World of Mathematical Equations.
- The Schrödinger Equation in One Dimension as well as the directory of the book.
- All about 3D Schrödinger Equation
- Mathematical aspects of Schrödinger equations are discussed on the Dispersive PDE Wiki.
- Web-Schrödinger: Interactive solution of the 2D time dependent Schrödinger equation
- An alternate derivation of the Schrödinger Equation
- Online software-Periodic Potential Lab Solves the time independent Schrödinger equation for arbitrary periodic potentials.


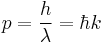
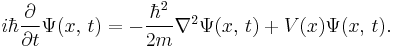

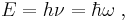
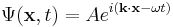
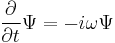
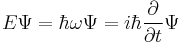
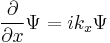
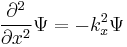

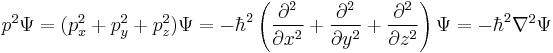
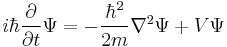
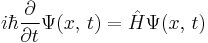
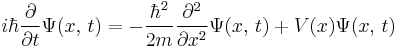

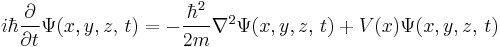
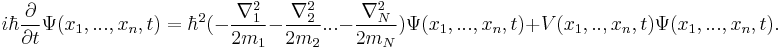
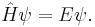
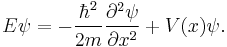

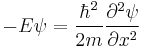


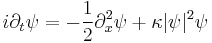
![H=\int \mathrm{d}x \left[{1\over 2}|\partial_x\psi|^2+{\kappa \over 2}|\psi|^4\right]](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/e1b35484450918d6b846dd70d8cb6bf9.png)